Mathematical Magnetism

Even though earth mankind of today was late coming to the party, Sir Isaac Newton brought to us improved clarity on gravity and its impact on earth matters and the solar system. The old example of an apple falling from a tree is used to demonstrate earth mankind’s step up in understanding of the fundamental force at work all around us.

Certainly, people of the much distant past recognized something like a bucket of stones was much harder to carry than a bucket of apples but it was just understood to be that way without a fundamental understanding of why. As we have grown in our understanding such as through Einstein’s Theory of Relativity, we seem to develop more questions than the issues we are explaining or beginning to understand. Quantum mechanics ushered in a whole host of complex issues and essentially separated the common person of high school education from the Ph.D. specialist. Our society has developed a serious wall between those with mathematical skills or scientific training from those who have to fix the contraptions developed by engineers to solve certain problems. The complexity wall has developed to the point where two diverse Ph.D. specialists cannot even communicate with each other, let alone to the masses.

It is very important for the communications to change. Most of the nations of earth are governed by some type of democracy where votes are tallied by the masses and not by specialists. It seems amazing that the USA can use the opinions of the masses to fund the research for NASA and other complex programs without getting into endless debates.

When we talk about forces, most of us think of a push or pull and maybe some understand the relationship of Force= mass times acceleration. We feel acceleration when we step on the gas of a powerful automobile or takeoff in a jetliner. Even the centrifugal force of a merry-go-round is a common example. We are generally familiar with unlike magnetic poles attracting and like poles repelling. But even technical people are far less aware of a magnetic force which “holds at a distance”. The force of gravity, electrostatic and simple magnetics simply pull all the way together or push as far as they can apart. There are no common examples of a force which “holds at a distance” and creates strongly held geometric patterns.

Some folks with a little bit of training in chemistry and physics know the theory of shells in the structure of atoms and molecules. When constructing increasingly more complex atoms, electrons are added in shells. Hydrogen and helium each have one and two electrons in the “1 shell”. The next more complex atoms starting with lithium up thru carbon, onto oxygen and finally to neon have up to 8 electrons in the “2 shell” making neon have 10 electrons in total. Strangely enough, not even the most specialized scientists have more than theories as to why this happens in the manner it does. Quantum mechanics provides a good basis for technical discussions for “what it is”, but does not address how it came to be.

Even more striking in our scientific ignorance is how light comes to happen. Most of what we use in light technology utilizes spectral analysis to study photons, the basic particle of light, emanating from an atom where the electron has gone from a higher energy state to a lower one. The wavelength of this light is super precise and allows us to study stars in far distant galaxies and know what their matter distribution is while we remain on earth.

All of us who have gone camping and had a campfire know that the fire puts out red to orange light enough to see to walk around in the night when near to the fire. Many others know that light from a very hot electric welding arc is much whiter and brighter. Similarly, street lights utilizing electron technology put out enough light to see far down the street at night.

But the actual mechanism for the development of light from atoms is far less known. From the complexity of quantum mechanics, we say that the electron position is simply a probability of existence and not an orbital position as taught for decades and still taught in initial scientific classrooms. The probability of something the size of a photon actually hitting something the size of an electron moving at tremendous speed and random direction is crazy crap.

Much more likely is the photon striking the magnetic field element holding the electron in structural connection and that field then increases its energy and the electron is then under different rules.

A physicist named David LaPoint has posted on You Tube a new theory about the interaction of all matter, including nuclei and electrons and furthered the theory with larger phenomena such as solar systems, galaxies and special bodies discovered by the Hubble Telescope. He has developed the magnetic principle of “magnetic force that holds at a distance” or holds in an organized position.

I have had a 50-year career as an engineering manager and have helped highly specialized technical people reveal their ideas to manufacturing managers with much less specialization, but who happen to be in charge of the funding for any research that is to be done. Mr. LaPoint is likely a Ph.D. physicist and has probably worked at CERN (European Organization for Nuclear Research), or someplace like it, and has had access to enormous amounts of observatory images and scientific communities such as CERN. It is likely that he is quite computer literate and certainly has access to computer graphics for making his presentations. It is very well done and should be viewed several times. One can click on the link below and see the first Primer Field, as he calls it. The length is about 54 minutes.

(update: Mr. LaPoint has resurfaced again and is continuing his work)

https://www.youtube.com/watch?v=9EPlyiW-xGI&t=70s

The important portion of his research is the discussion of how the magnetic field forces the balls into together and yet , he states, to the induced field in the balls, keeps them apart and into very precise patterns. What you cannot see in the video but shown herein is the super precise alignment of the balls and the precise relationships of both lengths, areas and angles as well as other mathematical relationships.

Note in the video that it starts with an image of dozens of smaller balls indicating a precise pattern that is only changed when additional balls are added. With just two balls, the pattern is like a dumbbell without connecting rod. With three, a perfect equilateral triangle, four is a square, five is a pentagon and so forth up to the pattern used herein with a double hex. Using the larger balls in the eye of the bowl magnets, note that when the first ball comes in that it overshoots its position and does a dance until landing on a precise location. If the ball were removed and then returned, it would go to the same location. This portion of the research is very systematic and mathematically precise.

When the second ball comes in, the first ball does a dance with it until they both settle on a new location. One can see that these are far from random locations. For a given camera location, all the patterns are highly repeatable in spacing and orientation.

For me, this is a solid indication, as Mr. LaPoint puts forth in the video, that the electron positions in atoms are likely the result of magnetic shells and that is why the wavelengths for a given atom are so mathematically precise and extremely repeatable. It also solves the problem of why only a certain number of electrons can occupy a given shell.

My contribution to the elucidation of this technology is to import specific images into ACAD engineering software packages and see if that analysis can shed any clarity as to what may be going on at a fundamental basis.

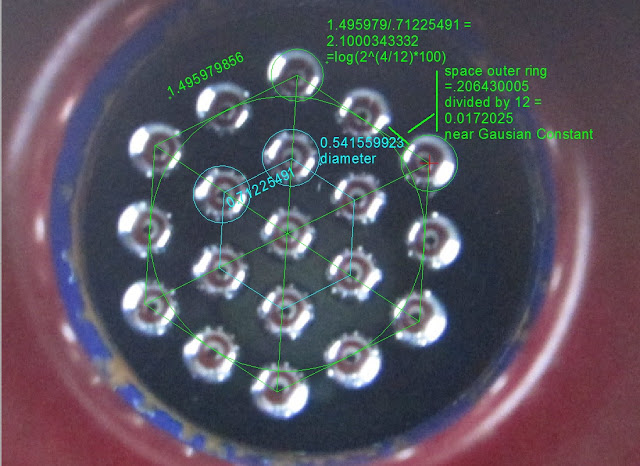
The image below is from one of David LaPoint’s oldest postings shows concentric hexagon patterns with 5 mm balls. There is a problem with the lighting which appears to show the center of the ball as a dark spot but is actually the reflection of the bowl down off-center to the ball. On the upper right cyan ball, the red cross-mark shows the actual center to be right over the point of the cyan hexagon. When the image is blown up in ACAD, it is clear where the actual ball is as minimally lighted surfaces become more visible.

In ACAD it is routine to change the scale to reflect any type of model that might have been discovered thru other means. However, dimensionless ratios reflect their design no matter what the scale is. The logarithmic ratio is particularly important because of its relationship to the twelfth root of 2.

The green hex side and radius is set to the Royal Cubit astronomical derivation at 1.495979856 and the dimensionless ratio with the cyan inner hex is log(2^^(4/12)*100) where log is to the base 10 and 2^^(1/12) is the half step musical scale used in all of earth human musical tuning from Middle A = 440 cps. This is the starting point for a whole series of mathematical relationships typically found in the LaPoint images.

(Royal Cubit AU relationship [20625/144)^^(1/2) / 8] = 1.495979856)

In the analysis in MathCad, Ax was set to various values near to

(1.495979856 x 10)^^(1/5) = 1.717849644 such “sidebig” became the Royal Cubit 1.4959xxx number.

log(2^^(1/3)*100)=2.100343332 sidebig/2.100343332 = sidesmall

5979856)

In the analysis in MathCad, Ax was set to various values near to

(1.495979856 x 10)^^(1/5) = 1.717849644 such “sidebig” became the Royal Cubit 1.4959xxx number.

log(2^^(1/3)*100)=2.100343332 sidebig/2.100343332 = sidesmall

(Royal Cubit AU relationship [20625/144)^^(1/2) / 8] = 1.495979856)

In the analysis in MathCad, Ax was set to various values near to

(1.495979856 x 10)^^(1/5) = 1.717849644 such “sidebig” became the Royal Cubit 1.4959xxx number.

log(2^^(1/3)*100)=2.100343332 sidebig/2.100343332 = sidesmall

The image below can be copied and blown up for clarity. It is from MathCad and represents the definition of sidebig and sidesmall from above. The ball diameter is 0.54156 instead of 5mm because other relationships develop and, in the scale, ACAD image, that is what the diameter of the ball is. In the near bottom line, one can see that the ball diameter easily converts to the exact average wavelength of the second most abundant helium and hydrogen isotope. Also in the bottom left, the Gaussian Constant is closely approximated.

The image below is a still from the LaPoint Primer Field #1 and demonstrates that there might be similar structure in the multi-rings of balls. The dimensionless distances where the camera is near perpendicular to the ball diameters indicates that there is some likely mathematical structure. The reciprocal of 0.72 = 1.3888 and the reciprocal of 0.15 is 6.6666. This seems to indicate that the number six continues to play some role in the structure with these particular magnetic pairs.

We know the immense complexity of organic chemistry develops from the carbon atom structure heavily influenced by hexagonal interconnected patterns. All living material owes its structure to the structure of carbon rings. For me, this indicates that all living matter is developed from the magnetic force that “holds at a distance”. It is the property of “induced magnetism” that is the primary influence.

Like a stone rolls down a hill to the bottom, the balance of the primary magnetic field of the bowls adjusts to the induced magnetic fields in the balls to form a sort of “low energy configuration” that is a precise pattern. The triangle, square and pentagon patterns have not been analyzed as of this posting.

The pair of stills below indicates how a change in the magnetic field of the larger bowls effects the location of the smaller bowl magnets. In the left picture, the large magnets are nearly together. In the right picture the large bowls have moved apart and that makes the smaller bowls roll out to a distance and held there. But in the video one must note that the smaller magnet doesn’t move away forever, but reaches a point of balance and locks into position. There is no other force known to me that holds objects at a distance and forms such precise patterns.

One can see in the image below of filings with a bar magnet that the sharp shavings are not free to move around like steel balls on a plastic sheet but there still is clear evidence that the pieces seek out a fairly uniform distribution and there are somewhat regular spacings between the lines of force. If one drags the filings out of alignment, they slip back into place.

Note that the shape of lines of force progress from the inner blue flat oval with horizontal the long axis and then up thru the red circle which is nearly a circle and out to the outer blue ellipse that has the long axis in the vertical. The bowl-shaped magnetic fields probably are more consistently one shape. The shape of the sun’s magnetic field is likely closer to circles and the eccentricity is a good indication of that. Also, the planets have a slightly different inclination

The sun’s magnetic field is quite complex and not fully mapped. One reason is that the mass ejections actually sends particles out through the solar system which to a large part is responsible for the geomagnetic field of the earth and some planets. It seems likely that magnetics plays an important role in the organization of the solar system.

There is no doubt in my mind that Mr. LaPoint’s efforts should be thoroughly studied and efforts made to better document them from a mathematical point of view. For my purposes, the simple demonstration of a potential organizing force is enough for now.

The Hexagon of Saturn

The image below is inserted here for an extension of what David LaPoint talks about in one of his videos.